October 30, 2004
Language, thought and counting in Amazonia
In the Oct. 15 issue of Science: two different groups of Amazonians, same basic result.
Peter Gordon's article on the Pirahã was pre-published online on August 19, and elicted a lot of comment at the time (Language Log postings here, here, here, here and here). His article's formal publication (in the October 15 issue) is matched with another report, this one on the Mundurukú:
Pierre Pica, Cathy Lemer, Véronique Izard, and Stanislas Dehaene, "Exact and Approximate Arithmetic in an Amazonian Indigene Group", as well as a
As Gelman and Gallistel put it,
The research findings indicate that, whether or not humans have an extensive counting list, they share with nonverbal animals a language-independent representation of number, with limited, scale-invariant precision. What causal role, then, does knowledge of the language of counting serve? We consider the strong Whorfian proposal, that of linguistic determinism; the weak Whorfian hypothesis, that language influences how we think; and that the "language of thought" maps to spoken language or symbol systems.
Gelman and Gallistel discuss the background of theories and experiments about language and thought in general, and language and numerical cognition in particular, and conclude that
reports of subjects who appear indifferent to exact numerical equality even for small numbers, and who also do not count verbally, add weight to the idea that learning a communicable number notation with exact numerical reference may play a role in the emergence of a fully formed conception of number. The challenge now is to delineate that role.
Unfortunately, if you don't have an a subscription to Science, you can't read what they have to say, nor can you read Gordon's work, nor the work of Pica et al. [Update: here is a .pdf of the Gelman and Gallistel viewpoint piece, and here is a .pdf of the Pica et al. article. You may be able to get a .pdf of the Gordon article here -- if that doesn't work, try clicking through from Peter Gordon's web page. See note at the bottom of this post for more links]
Peter Gordon's stuff has been fairly extensively discussed here already, so I'll just say a few words here about the Mundurukú. Here's where they live:
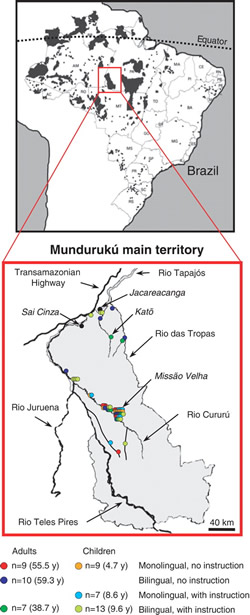
(A map showing where the Pirahã live can be found here.) The language of the Mundurukú is Ethnologue code MYU, with about 2,000 speakers, fairly close to Guraní with about 5,000,000 speakers. It has words for one, two, three and four, and "hand" is used for five or so:
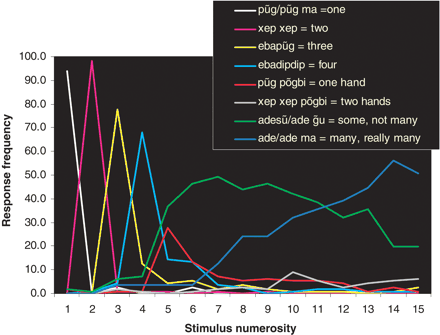
In estimating quantities, according to the article,
The Mundurukú did not use their numerals in a counting sequence, nor to refer to precise quantities. They usually uttered a numeral without counting, although (if asked to do so) some of them could count very slowly and nonverbally by matching their fingers and toes to the set of dots. Our measures confirm that they selected their verbal response on the basis of an apprehension of approximate number rather than on an exact count. With the exception of the words for 1 and 2, all numerals were used in relation to a range of approximate quantities rather than to a precise number. For instance, the word for 5, which can be translated as "one hand" or "a handful," was used for 5 but also 6, 7, 8, or 9 dots. Conversely, when five dots were presented, the word for 5 was uttered on only 28% of trials, whereas the words for 4 and "few" were each used on about 15% of trials. This response pattern is comparable to the use of round numbers in Western languages, for instance when we say "10 people" when there are actually 8 or 12. We also noted the occasional use of two-word constructions (e.g., "two-three seeds"), analogous to references to approximate quantities in Western languages. Thus, the Mundurukú are different from us only in failing to count and in allowing approximate use of number words in the range 3 to 5, where Western numerals usually refer to precise quantities.
I won't give the details of their experiments here, nor the background literature against which they interpret it. A description of some of their experimental techniques can be found in this 10/21 Guardian article by Brian Butterworth. However, I'll reproduce their conclusions, omitting the footnotes:
Together, our results shed some light on the issue of the relation between language and arithmetic. They suggest that a basic distinction must be introduced between approximate and exact mental representations of number, as also suggested by earlier behavioral and brain-imaging evidence and by recent research in another Amazon group, the Pirahã. With approximate quantities, the Mundurukú do not behave qualitatively differently from the French controls. They can mentally represent very large numbers of up to 80 dots, far beyond their naming range, and do not confuse number with other variables such as size and density. They also spontaneously apply concepts of addition, subtraction, and comparison to these approximate representations. This is true even for monolingual adults and young children who never learned any formal arithmetic. These data add to previous evidence that numerical approximation is a basic competence, independent of language, and available even to preverbal infants and many animal species. We conclude that sophisticated numerical competence can be present in the absence of a well-developed lexicon of number words. This provides an important qualification of Gordon's version of Whorf's hypothesis according to which the lexicon of number words drastically limits the ability to entertain abstract number concepts.
What the Mundurukú appear to lack, however, is a procedure for fast apprehension of exact numbers beyond 3 or 4. Our results thus support the hypothesis that language plays a special role in the emergence of exact arithmetic during child development. What is the mechanism for this developmental change? It is noteworthy that the Mundurukú have number names up to 5, and yet use them approximately in naming. Thus, the availability of number names, in itself, may not suffice to promote a mental representation of exact number. More crucial, perhaps, is that the Mundurukú do not have a counting routine. Although some have a rudimentary ability to count on their fingers, it is rarely used. By requiring an exact one-to-one pairing of objects with the sequence of numerals, counting may promote a conceptual integration of approximate number representations, discrete object representations, and the verbal code. Around the age of 3, Western children exhibit an abrupt change in number processing as they suddenly realize that each count word refers to a precise quantity. This "crystallization" of discrete numbers out of an initially approximate continuum of numerical magnitudes does not seem to occur in the Mundurukú. [emphasis added]
I'll take this as confirmation that my analogy to throwing was not completely nuts (though I certainly admit that language, internal or external, is likely to be more involved in the development and deployment of skilled arithmetic than in the comparable aspects of skilled throwing). "Language" and "thought" are not the only actors in this cognitive drama.
[Update: here are some videos of Mundurugú finger and seed counting, and a description of the videos.]
[Update #2: David Nash emailed that
..from your posting the description of Munduruku fits pretty well with what I understand to be a situation in Australian (Aboriginal) language communities, or at least as it was before schooling in numeracy...
and added that Bill McGregor is writing a review of Australian number classification. ]
[Update #3: Here is an excellent page discussing research on arithmetic and the brain at Stan Dehaene's Unité de Neuroimagerie Cognitive (Cognitive Neuroimaging Unit), with many links including to the new Science paper. ]
Posted by Mark Liberman at October 30, 2004 03:30 PM