May 30, 2007
Dediu and Ladd again
I've been waiting to read the paper by Dediu and Ladd ("Linguistic tone is related to the population frequency of the adaptive haplogroups of two brain size genes, ASPM and Microcephalin") that I mentioned a couple of days ago, but press coverage is increasing, and the paper doesn't seem to be up on the PNAS site yet. So I'll go ahead and make a some intitial observations based on their excellent "further information" site at Edinburgh, and my memories of a talk that Bob Ladd gave here at Penn a few months ago.
This research began (as I understand it) when Bob Ladd noticed that maps of the geographical distribution of two particular genetic traits (from a couple of articles published in Science in 2005) seemed similar to what he knew of the geographical distribution of lexical tone. So he worked with Dan Dediu to check this impression statistically. They looked at the geographical distribution of 26 linguistic traits (from Haspelmath et al.'s World Atlas of Language Structures) correlated with the geographical distribution of 1,000 genetic variants, yielding 26,000 correlations. The statistical distribution of these correlations looks like this:
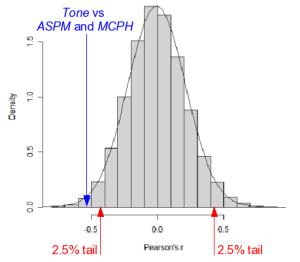
And sure enough, as the arrow on the graph indicates, the correlation between the geographical distribution of lexical tone and the two genes of interest is way out in the tail of this bell-shaped curve of empirical gene/language correlations. This clearly tends to confirm Bob's original intuition.
But even in a distribution created by completely random effects, without any meaningful connections among the variables studied, something has to be way out in the tail.
There's a tricky point of statistical reasoning here, which is clear in general, although even experts often seem to wind up disagreeing about particular cases. If you started with 26,000 correlations, and no reason to expect any causal relations among the variables, and a situation (like geographical diffusion of genetic and cultural traits) where it's not clear what distribution of correlations to expect in the absence of causal connections, then you probably wouldn't draw any conclusions at all from the fact that some of the correlations were fairly high. On the other hand, if you thought that there might be some meaningful connections in the mix, then the tails of the distribution are a good source of examples to study further -- and this is the main conclusion that D & L draw from their results, quite properly.
If there is some initial reason to suspect a connection between two traits -- for example, if D & L had started from the discovery that the alleles in question had a significant effect on the relative psychophysical salience of pitch vs. timbre -- then the discovery of a strong geographical correlation would be very strong result indeed. But in this case, what they started with was Bob's informal impression of a geographical correlation, and they confirmed this impression via a statistical demonstration that the correlation in question is indeed among the largest 1.4% of 26,000 correlations tested, i.e. among the top 360 or so. This proves that Bob Ladd has a good eye -- but have they in effect evaluated one trial of one hypothesis, or some much larger number of hypotheses and/or trials, as high as 26,000? Presumably it's somewhere in between.
An analogy may help to clarify this point. If I claim to have a trick (or lucky) penny, and we try it out together, and discover that it comes up heads 9 times out of 10, we'll conclude that my claim is probably valid. Theory aside, we could do this empirically by reference to a database of coin-toss experiments, using a wide variety of randomly chosen pennies, in which we find that 9 out of 10 heads or better only occurs about once in 100 trials.
But suppose, instead, someone points out to us that on a given Saturday during football season, in a collegiate league whose teams play in 10 games, 9 out of 10 of the referees' coin tosses came up heads, and asserts on this basis that the refs are crooked. Should we accept the argument? Probably not, since we're only checking this case because it struck someone as unusual. [More on this issue, in a more frivolous context, here.]
Just to underline one aspect of this discussion, I did a trivial little simulation, in which individual mutations were placed at random on a 20x20 grid, and then died, reproduced and migrated at random for 35 generations. (The grid was configured as a manifold, so that if you migrate off of one side, you come back in on the opposite one.) I ran 100 traits independently, and then looked at the geographical correlations among their population frequencies:

(The number of correlations was actually less than the expected 4,900, because when a trait vanished from the population before the end of the simulation, I didn't include it in the final calculations. 26 out of 100 traits went extinct, so the number of correlation in the plot above is actually .5*74*74-74 = 2,664. This is also the reason for the preponderance of higher positive correlations over negative ones, I believe, since unusually successful traits tend to have a high frequency everywhere, whereas unusually unlucky ones simply vanished. The larger number of small negative correlations is (I think) the natural consequence of limited diffusion from geographically-random point mutations.)
I suspect (though I haven't shown) that by jiggering the parameters of the simulation, you could get a frequency-distribution of geographical correlations rather like the one the Dediu and Ladd found, without assuming any meaningful connection at all between genes and linguistic traits. And of course there really are are some connections, if only because of linguistic endogamy.
The point is not in any way to debunk Dediu and Ladd -- I don't think that they would disagree with what I'm saying here, and I certainly don't disagree with their conclusions, as I understand them from what I've read so far. Their results are suggestive, and well worth following up. Indeed, I think that the other few hundred top-ranked gene/language correlations should be investigated as well!
But unsurprisingly, the press response is full of headlines like "Speaking in tones? Blame it on your genes" and "Genes may help people learn Chinese". It's worth noting that if there's a causal connection here, it need not have anything to do with the relative ease of learning tonal distinctions. In the talk that he gave here, Bob Ladd speculated that perhaps there is a difference in the relative psychophysical salience of the "micromelodies" that are a universal and inevitable consequences of consonant and vowel articulations in every language, leading to differences in the propensity to create tonal contrasts by re-interpretation of segmental contrasts, as happened repeatedly in the reconstructed history of East Asian and Meso-American tone languages.
[Update -- a response by Ladd and Dediu is here. The PNAS paper is here.]
Posted by Mark Liberman at May 30, 2007 12:03 PM